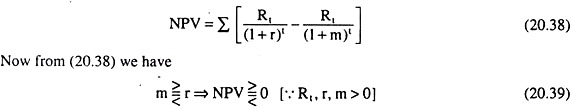Comparison between the Project Evaluation Methods of NPV and IRR:
The NPV and IRR methods of project evaluation seem to be consistent with one another. For, if a project is considered to be acceptable or not according to one of them, it would be considered in the same way by the other method also.
From equations (20.34a) and (20.37a), we obtain:
It is evident from (20.39) that if a particular project is accepted or rejected (m ≠ r) by the IRR method, then it would also be accepted or rejected (NPV ≠ 0) by the NPV method. And if the firm is neutral between accepting or rejecting (m = r) the project by the IRR method, then it would also be neutral (NPV = 0) in the same way on the basis of the NPV method.
ADVERTISEMENTS:
However, in the case of selection of one investment project out of two or more alternative projects, the NPV and IRR methods may give contradictory signals, i.e., if the NPV method gives a project the rank of one or two, the IRR method may assign the rank of two or one to the same project. That is, out of two different projects, if one is to be selected by the NPV method, the other would be selected by the IRR method.
This is because of the fact that the two methods make different assumptions on the rate of return that is obtained from the reinvestment of the annual cash-flow that the firm would have from any particular project. The NPV method implicitly assumes that reinvestment would take place at the rate of cost of capital. The IRR method, on the other hand, assumes that the reinvestment would take place at the IRR itself.
Owing to this difference in the rate at which reinvestment would be made, the NPV and the IRR methods may give different evaluations of two different projects if:
ADVERTISEMENTS:
(i) The expected life of the projects be different, and/or
(ii) The initial costs of the projects be different, and/or
(iii) The cash-flow pattern to be obtained from the projects be different.
We may note here that the assumption regarding the rate at which the reinvestment of cash-flow is to be made in the NPV method is theoretically more sound than that in the IRR method. Because, the NPV method applies the same rate (the rate of cost of capital) at which reinvestment is to be made, to each different project.
ADVERTISEMENTS:
The IRR method, on the other hand, assumes that a project with a higher IRR would have a higher rate of return from the reinvestment and that with a lower ERR would have a lower rate of return from the reinvestment. Since the cash-flow patterns are different in different projects, the rates of return from reinvestment are obtained to be-different in different projects in the IRR method.
These rates would be the same only in those projects for which the IRRs are also the same. Because of this inconsistency of the IRR method, the NPV method is considered to be more acceptable than the IRR method.