The basic theme of the classical model was the development of the economy from a progressive state into a stationary state. However, “the ultimate arrival, at which wages would have reached a minimum acceptable level and net investment would have ceased because of low profits, could be postponed indefinitely by a stream of highly productive inventions.”
The classical theory is basically a synthesis of the doctrines put forward by Adam Smith, T. R. Malthus, David Ricardo, J. S Mill and others.
The following classical propositions are worth mentioning in this connection:
1. According to the classicists, one central feature of the progressive state was a high level of accumulation, which permitted an increase in the output of the community by raising labour productivity (as also that of land) by adding to the productive resources available. The rate of accumulation basically depended on the level of profit.
ADVERTISEMENTS:
2. Total profits depended on two primary factors: the total product of labour, and the level of wages. As a corollary, it also depended on the marginal productivity of labour.
3. The productivity of labour depended on the stock of capital as also the technique employed.
4. In the short run actual or market wages could lie above the subsistence level which would warrant an increase in population. But in the long run, due to population growth, wages tended to approach the subsistence level. And, as a result, population growth would come to a halt.
The classical model may be summed up in terms of the basic Baumol- diagram. To start with, we assume that in the early stage of the classical economy population is small compared to natural resources. Consequently, profits the rate of accumulation, and thus wages are all relatively high. It is also assumed that population adjusts itself relatively quickly to a change in the level of market wages (the wages actually paid in practice).
ADVERTISEMENTS:
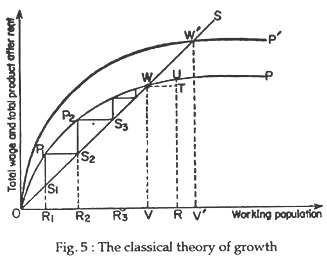
In Fig. 5, the total wage payments, if subsistence wages were always just paid, would be given by a straight line OS through the origin. The slope of OS (VW divided by OV) is thus “total subsistence wage payments divided by the size of the working population, i.e., the wage necessary to keep one person at the minimum standard.”
Here, the OP curve gives the total product of the economy after rent payment. This curve is convex upward due to a twofold reason: the operation of the law of diminishing returns and rise in rent payments with population growth.
Thus, for instance, when population increases from zero to OR1, total product after rent payment increases by R1P1. But, when working population is higher than an equal increase in population, this time from OV to OR, allows only a small increase in product (TU here).
ADVERTISEMENTS:
Let us start from a population OR1. If wages are pegged at the subsistence level total wage Payments Would be R1S1 and an amount S1 P1, the difference between total product minus rent and total will be left over for profit. This will lead to capital accumulation.
As a result wages will be pushed up to R1P1 since there is high demand for labour in an expanding economy. But once this wages is reached the surplus of the capitalist disappears altogether since workers eat up the entire product. And accumulation ceases.
This is thus a position of temporary equilibrium. However, high wages will induce population growth. This will go on until wages are again driven down to subsistence level, i.e., population will rise to OR2. Here again there will be profits, given by S2P2, so accumulation will begin again.
Wage payments will then be driven up to R2P2, and population will grow to OR2, and so on. In a series of steps represented by the stepped line S1P1S2P2S3 …………, this gradually approaching the point W where the two curves OP and OS intersect.
At point W there will be no profits even with wages at subsistence level, VW, since these wages will exhaust total product minus rent. There would thus be no motive for further accumulation and population will remain stationary at OV. The classical stationary will have been reached.
In this condition, the mass of mankind is generally miserable. Only the landowner would be better off. The increase in population has made land very scarce and would have raised rents considerably. Technological changes or innovations may cause a shift in the productivity curve (from OP to OP’ in the diagram) and postpone the day of judgment but cannot help matters much.