Quotas and Tariffs Imposed by the Government

Let us make an in-depth study of the important quotas and tariffs imposed by the government.

Many countries use import quotas and tariffs to keep the domestic price above world levels and, thereby, enable the domestic industry to enjoy higher profits than it would be possible under free trade.

We will see that the cost to society from this protection can be very high, where loss to consumers exceeds the gain to domestic producers.

Without a quota or tariff, a country will import a good when its world price is below the market price that would prevail if there were no imports. Fig. 8.26 shows this — S and D are the domestic supply and demand curves. Without imports, the domestic price and quantity would be P_O and Q_O which equate demand and supply.

But world price P_w < P_O, so domestic consumers would be in a position to buy from abroad at a lower price in a free trade situation. The import will reduce the domestic price to the level of world price, P_w, and, at this lower price, domestic production will fall to Q_d, and the domestic consumption will rise to Q_T. Imports are then the difference between domestic consumption and domestic production, Q_T – Q_d.

Now, suppose, the government restricts imports by imposing quota of zero. What are the gains and losses from such a policy?

Without any imports, the domestic price will rise to P_O. Consumers who purchase the good will pay more and lose an amount of surplus given by the areas A and B. Also, given this higher price, some consumers will no longer buy the good, so there is an additional loss of consumer’s surplus, given by the area C. The total ∆CS = – A – B – C.

What about producers? Output is now higher and sold at a higher price. Producer’s surplus, thus, increases by the areas A. ΔPS = A. The change in total surplus, ΔCS + ΔPS = – (B + C). Again, there is a deadweight loss — consumers lose more than producers gain.

Imports could also be reduced to zero by imposing a large enough tariff. The tariff would have to be equal to or greater than the difference between P_O and P_w. With a tariff of this size, there will be no imports and, therefore, no government revenue from tariff collections; so, the effect on consumers and producers would be the same as with quota.

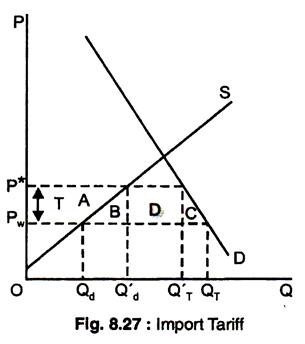
More often, government policy is designed to reduce — and not to eliminate — imports. Again, this can be done with either a tariff or a quota as in Fig. 8.27. With free trade, domestic price will be equal to the world price and imports will be Q_T – Q_d. Now, suppose, a tariff of £T per unit is imposed on imports and this will rise domestic price to P* and domestic production but domestic consumption will fall.

In Fig. 8.27 we can see the loss of consumer’s surplus given by A + B + C + D and producer’s surplus as A. If a tariff is used, the government gains D — the revenue from the tariff, so the net domestic loss is B + C. If a quota is used, area D becomes part of the profits of foreign producers, and net domestic loss is B + C + D.