Dynamic Multiplier Action | Macro Economics

The below mentioned article provides notes on Dynamic Multiplier Action.

That is, we started with a macroeconomic equilibrium situation and asked what would be the new equilibrium corresponding to a change in net investment of a given amount, and how the new equilibrium would differ from the original one.

In the actual world adjustment as a result of change in net investment takes time.

Therefore, to get a more complete picture of the events connected with a change in net investment, we have to follow the path of movement from one equilibrium situation to another equilibrium situation. An enquiry into the process of movement requires the treatment of multiplier problem in terms of dynamic analysis.

In other words, the analysis of a single investment shock can be easily extended to cover the analysis of effects over time of a permanent change in net investment. A permanent increase in net investment is a continuous series of investment shocks of equal size.

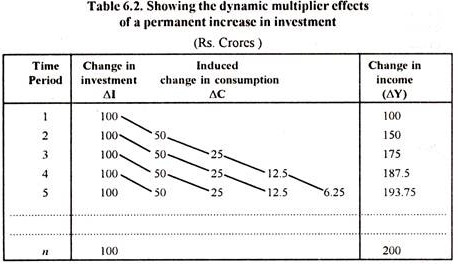
Let the initial equilibrium be disturbed by a permanent increase in net investment of Rs. 100 crores per period of time. The level of investment now exceeds the original level Rs. 100 crores not only in period 1 but also in all the following periods. In each period an investment of Rs 100 crores now takes place. We simply add the effects over time of the individual investment shocks to obtain the total effect of the rise in the level of net investment.

Let us further assume that all trans-actors have a mpc of 1/2 and that they react to a change in income in any one period by changing consumption expenditure only in the following period. On these assumptions, the process resulting from a permanent increase in net investment of Rs. 100 crores per period is as shown in the table:

In period 1 income rises by Rs. 100. In period 2 this increase in income leads to an increase in consumption of Rs. 50 and thus to a rise in income above the original level of Rs. 50. But in period 2 there is again additional investment of Rs. 100, and thus a further increase in income of Rs. 100, so that the total increase in income (over the original level) in period 2 is Rs. 150.

In period 3 the rise in income is Rs. 175, including an additional investment of Rs. 100 in period 3. But increased consumption expenditure is Rs. 50 (resulting from the increase in income due to increase in investment in period 2) and of Rs. 25 increased consumption expenditure (resulting from the increase in income due to the increase in consumption expenditure in period 2) and so on, as shown in the table.

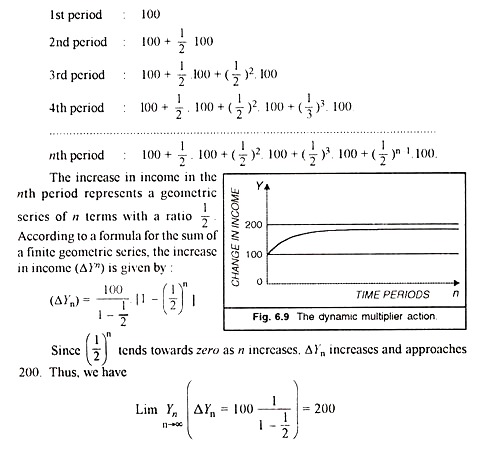
The arrow pointing from the investment of one period shows the effect of a single investment shock. The row for any one period contains the components of the increase in income during that period, and shows from which investment shock the individual components derive. With a mpc of 1/2 , the increases in income in the successive periods are shown by the following sequences.

The figure 6.9 and the table given earlier show the increase in income ∆Y_n as n increases. With a mpc of 1/2 the final effect of a permanent increase in net investment of Rs. 100 is a permanent rise in national income of Rs. 200. The curve in the figure shows the path by which this increase is finally attained. As can be seen, the final effect of the multiplier process is achieved only after an infinite number of periods ; in actual practice, however, the new higher equilibrium level of income is reached after a few periods (in our example it happen after five periods).

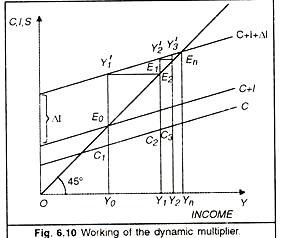
In the graphical representation of the working of dynamic multiplier given here, we assume that consumption depends on the income of the preceding period, C = C’’ faY _t-1 where a is the marginal propensity to consume and also that investment is a function of income and an autonomous constant I = F(Y, B), In the figure 6.10, consumption, investment and savings arc measured along the vertical axis and income is measured along the horizontal axis—all magnitudes being in real terms.

Thus C curve relates consumption to the income of the preceding period and it has a constant slope of 0.5. The original investment schedule is a vertical distance between the C curve and the C + I curve, while the new investment schedule is that between the C curve and the C +1 + ∆I curve, which gives a constant level of investment equal to ∆I. Saving out of previous income is measured by the vertical distance between the 45° line and the C curve. The system is initially settled at equilibrium point E₀ where saving and investment are equal (E_oC₁ = E₀C₁). This is our starting point.

In period 0, before the change, consumption is C₁Y₀ and income OY₀ or E₀ Y₀ thus leaving E₀C₁ both as saving and investment. Now investment increases by ∆I from period 0 to period 1 and stays at this level. What is the effect of this? Consumption remains unchanged because consumers have not yet had an opportunity to adjust themselves to change.

Similarly, intended savings remain unchanged. This means that investment exceeds intended savings by an amount equal to the increment of investment, or by Y₁E₀. This excess of investment over intended savings for period 1 (equal to actual savings for period 0) account for the unexpected increment of income from period 0 to period 1.

In period 1, there is an equal horizontal change. Y₁E₀ (= Y₀Y₁), for the vertical change of Y₁E₀. In other words, income increases over OY₀ by the amount of excess investment in period 1 after the injection of ∆I. The total income received in period 1 is therefore OY₁ (=OY₀ + K₀ Y₁) What would happen to saving investment relation in this period? Investment is again I + AI or Y₂C₂ and savings corresponding to previous income OY₀ arc E₁C₂, thus making intended savings fall short of intended investment by Y’₂E₁ (= Y’₂C₂ – E₁C₂). This excess investment is what causes income to increase from period 1 to the next, that is, by Y₁ Y₂.

Though consumption increases from C₁Y₀ to C₂Y₁ corresponding to the increased total income of OY₁ this extra consumption amounts to half of extra income Y₀Y₁. Similarly, saving increases by half of extra income Y₀Y_1. This explains why intended savings in period 1, fall short of investment. As such income expands by an amount equal to the above excess of investment over intended savings, or by Y₂E₁ which amount is equal to the horizontal change of Y₂E₂.

It is thus that period 2 is ushered in and total income received is OY₂. But investment in this period still exceeds saving by Y’₃E₂ (= Y’₃C₃ – E₂C₃). Since intended saving corresponding to previous income OY₁ is Y’₃E₂ short of a total investment of Y’₃C₃. This excess of investment over intended saving in period 2 leads to an increase in income from period 2 to period 3, and so on.

It may be noted that the gap between intended saving and intended investment (the vertical distance between the C +1 +∆I curve and the 45° line) becomes smaller over time, i.e., the increment of income which it produces becomes smaller and smaller until the gap is completely wiped out. The constant stream of new investment has developed its full effect and aggregate income has reached the stationary higher level of Y_n in the nth period. The economy has become stable at the new and higher equilibrium point E_n. Income has reached there via E₀, ,Y’₁,E₁, Y’₂,E₂…………………… E_n.

The multiplier has done its job, for it has caused income to expand and intended savings to expand upto the new level of investment; that is, where S_n = 1_n. Thus, in the nth period the saving gap (the vertical distance between the 45° straight line and the C curve at Y_n) equals the sum of old investment plus new investment (the vertical distance between the C + I + ∆I curve and the C curve at Y_n).

Qualifications:

We must note the following qualifications to the simple process of working of the dynamic multiplier:

(i) Allowance has to be made for the possibility that the mpc may not remain constant as income increases through the multiplier action.

(ii) However high the mpc may be, output and employment will stop growing once full employment has been reached. Beyond that level a further increase of investment would merely increase general prices.

(iii) The assumption of a given increase of investment, though useful, may have to be dropped in order to allow for the possibility that the multiplied income may also, in turn, induce fluctuations in investment.

(iv) Allowance must be made for the possibility that a part of the increased income may leak out to the benefit of foreigners, as far as open system with foreign trade relations is concerned.