Criterion of Welfare (With Diagram)

Criterion of Welfare # 1. The Parisian Criterion:

Pareto was the first economist to find out an objective test of social welfare maximum. Often called Pareto optimality, Pareto unanimity rule, Paretian optimum, social or general optimum, the Paretian criterion states that welfare is said to increase (or decrease) if at least one person is made better off (or worse off) with no change in the positions of others.

In other words, any change which harms no one and which makes some people better off (in their own estimation) must be considered to be an improvement.

Given certain rules of distribution, any economic reorganization is said to increase social welfare, if the welfare of some persons is increased without any decrease in the welfare of others. In terms of indifference curve analysis, an optimum position is one in which it is not possible to put any person on a higher indifference curve without causing someone to drop to a lower one.

By defining a welfare position in terms of ordinal utility, Pareto rejected the notion of cardinal measurement of utility and by so doing he dispensed with the need for interpersonal comparison of utility. Let us explain Pareto’s criterion with the help of utility possibility curve, as in Fig. 1

Suppose there are two individuals A and В sharing a given bundle of good X. A’s utility is represented on the horizontal axis and B’s utility on the vertical axis. Thus BA represents utility possibility curve of all combinations of the individual utilities.

The Paretian criterion shows that any change which causes a movement from С to F on the production possibility curve BA is an improvement because it makes both individuals better off thereby maximising their welfare.

Similarly, a movement from С to D or E on the BA curve is an improvement for it makes at least one person better off without making the other worse off. But any point outside the segment DE is not a Pareto improvement. For instance, any movement from С to H increases B’s welfare at the expense of A’s welfare.

Its Criticism:

The Pareto criterion, as pointed out by Dr. Graaf, is free from making any interpersonal comparisons. It is based on a very broad ethical positive view that ‘one should always do well to all’. But it has its weaknesses.

(1) There can be an infinite number of Paretian Optima, each with a different level of welfare. As pointed out by Pareto himself, there are an “infinite number of points at which maxima of individual optimalities are attained.” This criterion ex- plains little or nothing as to how it can be determined, whether one optimum position is better or worse than another optimum position. To take Boulding’s smile of the Paretian criterion ‘as a mountain having many peaks’.

If ‘height’ denotes welfare, each peak represents an optimum position. If one were to choose the highest peak, it involves interpersonal comparisons. It is, therefore, not possible to find out the best of the optimum points of welfare.

According to Dr. Dobb the “so-called Pareto-conditions are quite insufficient to characterize a position as optimum. A situation where they are fulfilled may well be inferior to a number of other situations where they are not fulfilled.” Strictly speaking, each Paretian optimum is sub- optimal. The Pareto optima are, thus, comparatively unimportant, accordingly to Dr Little.

(2) Not Possible to Judge Many Policy Proposals. There are many policy proposals which cannot be judged with the help of this criterion. It does not apply to any policy proposal which benefits some and harms others. According to Baumol, “The Pareto criterion works by sidestepping the crucial issue of interpersonal comparison and income distribution, that is, by dealing only with cases where no one is harmed so that the problem does not arise.”

(3) The Paretian Criterion is not free from Value Judgements. To say that it is possible to make every person better off without making any other person worse off is a value judgement in itself. Though Pareto used the method of ordinal measurement of utility, yet he could not present a value-free criterion.

(4) Pareto evaluates only Unambiguous Changes in Welfare. In his efforts to avoid interpersonal comparisons, Pareto evaluates only ‘unambiguous’ changes in welfare. The Paretian welfare criterion is, thus, of little use in making policy recommendations. For instance, in terms of Figure 1, a movement from С to any points on the segment DE is not always an unambiguous increase in welfare.

There may be other optimum welfare positions on the utility possibility curve BA outside DE.

According to Boulding, there are two types of changes in social welfare:

(i) Those which benefit all or at least one person through trading;

(ii) Those which benefit one person at the expense of the other through conflict. The Paretian criterion relates to the welfare optimum reached through trading whereby all or at least one person becomes better off without making any other person worse off. But this view is unrealistic, for all economic policies in a way benefit some persons and harm others. Thus the Paretian criterion lacks universal validity and renders welfare economics useless and sterile.

Conclusion:

Barone in his efforts to make the Paretian criterion realistic introduced the idea of a compensating payment. According to him, a change that makes one person better off and other worse off can lead to his economic welfare, if the gainer compensates the loser so that each returns to his original welfare position. But neither Barone nor Kaldor and Hicks after him, insisted on making actual payments. Scitovsky did suggest compensating payments being made actually. But all these efforts have not helped the welfare economists in evaluating policy changes on purely positive premises contrary to the Paretian criterion.

Criterion of Welfare # 2. The Compensation Criteria:

The compensation criteria also known as the New Welfare Economics have been formulated by Hicks, Kaldor and Scitovsky. Accepting Pareto’s ordinal measurement of utility and the impossibility of its interpersonal comparisons, they tried to show that social welfare could be increased without making value judgements.

The Kaldor-Hicks Criterion Assumptions:

The compensation criterion of Kaldor-Hicks is based on the following assumptions:

(a) Each individual’s satisfactions are independent from the others so that he is the best judge of his welfare.

(b) There is the absence of external effects in production and consumption.

(c) The tastes of each individual are constant.

(d) It is possible to separate the problems of production and exchange from the problem of distribution.

(e) It is assumed that utility is measured ordinarily and interpersonal comparisons are impossible.

Explanation:

According to Kaldor, the test of increase in social welfare is that if some people are made better off and others worse off, the gainers from the change could more than compensate the losers, and yet be better off them. The actual payment of compensation is regarded as a political or ethical decision. Kaldor does not require that the losers should actually be compensated. Rather, he requires that the gainers should be able potentially to compensate the losers out of their gains.

Hicks presents the same criterion in a little different way thus: “If A is made so much better off by the change that he could compensate В for his loss, and still have something left over, then the reorganization is unequivocal improvement.”

Thus the Kaldor-Hicks criterion implies that if an economic change leads to the production of more goods and services they can be so distributed as to make some people better off and none worse off. Actual redistribution, being a political or ethical issue, need not take place. It is enough that reorganization creates such conditions that a redistribution can be effected.

This criterion can be illustrated with the help of utility possibility curves for two individuals. If A and В are two individuals, each utility possibility curve represents the locus of all combinations of their utility levels. Each curve is related to a given fixed bundle of goods and the various points on each curve are obtained by costless lump sum redistribution of a fixed commodity bundle.

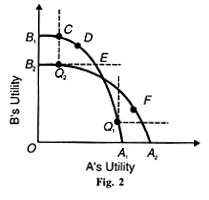
Let X and Y be the two bundles of goods represented by the utility possibility curves B₁A₁ and B₂A₂ respectively as shown in Figure 2 Starting from a given bundle of goods represented by Q₂ in terms of the Paretian criterion any change which leads to a movement to any one of the points C, D or E is a Pareto improvement on the B₁ A₁, curve because it makes both individuals better off or at least one better off without making the other worse off.

But any movement outside С and E to Q₁ cannot be evaluated by the Paretian criterion because it improves A’s welfare at the expense of B. However, a move from Q₂, to Q₁ can he evaluated in terms of the Kaldor-Hicks criterion. This can be done by

(i) Asking В how much he would be willing to pay to A to prevent this move, and

(ii) Asking A how much he would be willing to pay to В to forgo it.

If (ii) > (i), the change increases welfare because A would potentially compensate В for his loss and still be better off at Q₁ than at Q₂ A simple test for an improvement of welfare according to the Kaldor-Hicks criterion is that the initial bundle should He below the utility possibility curve representing the new bundle. Thus a move from O₂, to Q₁ satisfies the Kaldor-Hicks criterion, because Q₂ lies below the utility possibility curve В_t A_: of the final bundle Q_1. To present it differently, a move to Q₁ can be contemplated to generate the point D on the same utility possibility curve B₁A₁ which is unambiguously better than Q₂ After compensation one can move from D to Q_1.

Its Criticism:

This compensation criterion has been criticised by Scitovsky, Baumol, Samuelson, Little and others.

1. Ignores income distribution:

The Kaldor-Hicks compensation principle, according to Dr. Little, is merely a definition and not a ‘test’ of increase in welfare because it ignores income distribution. In fact, the problem of distribution cannot be ignored where the problem of productive efficiency is involved. To say that one ‘bundle of goods’ is greater than the other is meaningless without reference to income distribution. For any comparison between two bundles of goods involves their money values at their market prices.

2. Measures only potential welfare:

In trying to separate production from distribution, this criterion confuses potential welfare with actual welfare. It simply measures potential welfare changes associated with changes in any particular bundle of goods.

The actual welfare depends not only on the production of goods and services but also on their distribution. The compensation principle errs in neglecting the distributional aspect. It measures only the potential welfare which does not serve any practical purpose.

3. No common standard of value:

Prof. Baumol opines that when more than two commodities are involved, optimum production is not possible unless there is a common standard of value for measuring different commodities. But such a standard depends on income distribution which the compensation principle neglects. In such a situation, says Prof. Baumol, one has to use a standard that ‘bends and stretches and falls to pieces in our hands.’

4. Not free form Interpersonal Comparisons:

Kaldor, Hicks and their followers failed in their efforts to find out a value-free criterion. The Kaldor-Hicks criterion is based on the assumption that the ‘social value of money’ is the same in the hands of both the rich and the poor. Moreover, money is not actually transferred but remains with the better off.

Thus this criterion has a utilitarian scheme of ethics and involves interpersonal comparisons of utility. In fact, as pointed out by Dr. Nath, the attempts of Kaldor and others who followed him were foredoomed as “no prescriptions can be derived without starting from some ethical premises.”

5. Based on Long-Run Welfare Adjustments:

Little and Scitovsky have criticised Hicks for suggesting long-run welfare adjustments which would have insignificant real income distribution effects. Moreover, the effects would be random so that they cancel out in the long-run.

Scitovsky agrees with Little that some of the changes which might pass the Kaldor-Hicks criterion will have quite sufficient real income distribution effects, so that it would be, at best, wishful thinking to suppose that they would cancel out with the effects of other changes. If, however, the time period is long enough, even the people who are better off would be dead and this criterion then becomes meaningless.

6. Does not Involve Actual Compensation:

This criterion does not take into consideration the payment of actual compensation. It recognises only potential compensation with which actual increase in welfare cannot be measured. There- fore, actual compensation is necessary so that no individual remains a loser. But the payment of actual compensation involves many administrative problems which render this criterion impracticable.

7. No Universal Validity:

Scitovsky has criticised Kaldor for the view that the state is fully responsible for maintaining an equitable distribution of income. If there is unequal income distribution in a community, it is corrected as a matter of course by the state through a system of compensations.

According to Scitovsky, “This is likely to be the case in a socialist economy.” But in a free enterprise economy, the effects of a certain economic reorganization on efficiency and equity cannot be separated because compensation payments are not feasible politically. Thus the Kaldor-Hicks criterion has no universal validity, according to Scitovsky.

The Scitovsky Double Criterion:

Prof. Scitovsky has pointed out that the Kaldor-Hicks criterion leads to a contradiction. The Kaldor-Hicks criterion shows that a movement from Q, to Q_} is an improvement through redistribution in Figure 2. But if compensation is actually paid, it may lead to a different redistribution of income before and after the change. It is, there- fore, possible to have the reverse movement in terms of the Kaldor- Hicks criterion. In such a case, each situation would be better than the other.

This is the contradiction in the Kaldor-Hicks criterion, known as the Scitovsky Paradox. This can be understood with the aid of Figure 2 If both individuals A and В are at Q₂ then Q₁ which is better for A and worse for В is an improvement because from Q₁ both can go to D on the same utility possibility curve by means of redistribution which is a better situation than Q₂.

Similarly, it can be argued that Q₂ is better than Q₁ because from O₁ redistribution can lead to F on the utility possibility curve B₂A₂ which is a better situation for both A and B. Thus the two situations Q₁ and Q₂ would be better than the other which is a contradiction. This is known as Scitovsky’s reversal test.

To overcome this contradiction, Scitovsky suggests that a change is said to increase welfare if (a) the Kaldor-Hicks criterion is satisfied, i.e., the gainers are able to compensate the losers, and (b) the losers are incapable of bribing the gainers for not accepting the change. Thus Scitovsky’s double criterion requires the fulfilment of the Kaldor-Hicks test and the non-fulfillment of the reversal test. Therefore, a movement from Q₂ to С is an improvement because both A and В can move further to D where they are better off, but not from С to Q_1.

Its Criticism:

The Scitovsky double criterion is also not free from criticism:

1. It operates on the basis of an implicit and unacceptable value judgement. It thus avoids the basic problem of interpersonal comparison which is required to evaluate a policy change which harms one individual at the expense of the other.

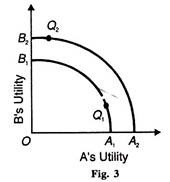
2. The Scitovsky criterion does not solve the problem arising from the Kaldor criterion even if the utility possibility curves do not intersect, as in Figure 3. At point Q₂, В is better off but A is worse off than at point Q₁. Even though the two utility possibility curves do not intersect, we come to the same conclusion reached by both Kaldor and Scitovsky criteria because the B₂ A₂ utility possibility curve lies above the B₁A₁ curve.

The Little Criterion:

Little does not consider the Kaldor-Hicks criterion and the Scitovsky criterion as satisfactory criteria of welfare. He is in favour of actual rather than potential changes in welfare and proposes his own criterion in these words: “A change is economically desirable if it results in a good redistribution of welfare, and if a policy of redistributing money by lump sum transfers could not make everyone as well-off as they would be if the change were made.”

Conclusion:

Thus, the various compensation criteria which led to the so-called New Welfare Economics were attempts at presenting a universally valid criterion for increase in welfare. These attempts, however, failed because the economists were not successful in offering a value-free welfare criterion.

Criterion of Welfare # 3. The Social Welfare Function: The Bergson Criterion:

The concept of social welfare function was first introduced by Prof. Bergson and later on developed by Samuelson, Tintner and Arrow. They are of the view that no meaningful propositions can be made in welfare economics without introducing value judgments. The concept of social welfare is an attempt at providing a scientifically normative study of welfare economics.

A social welfare function shows the factors 011 which the welfare of a society is supposed to depend. Bergson defines it “as a function either of the welfare of each member of the community or of the quantities of products consumed and services rendered by each member of the community.”

In its original form the Bergson social welfare function is formulated in a completely general manner. It is a function which establishes a relation between social welfare and all possible variables which affect each individual’s welfare, such as a services and consumption of each individual. It is an ordinal index of society’s welfare and is a function of individual utilities. It is expressed as

W = F (U₁,.U₂, U_n)

where W is the social economic welfare, F is for function, and U_r U₂……………… U_N is the levels of utilities of 1, 2,…individuals. W is an increasing function of these utilities.

The general properties of the social welfare function are similar to those of an individual utility function. In particular, the value of the welfare index increases whenever the utility level of one individual is increased without lowering that of the other individual. Thus the social welfare function is consistent with the Pareto optimality criterion, but it goes much further, since it assigns a value to every economic state, including those which according to the Pareto criterion are regarded as non-comparable. The existence of a social welfare function, therefore, implies a comparison of the welfare position of the individual members of society.

Assumptions:

The Bergson social welfare function is based on certain assumptions:

(a) It assumes that social welfare depends on each individual’s wealth and income and each individual’s welfare depends, in turn, on his wealth and income and on the distribution of welfare among the members of the society.

(h) It assumes the presence of external economies and diseconomies with their consequent effects.

(c) It is based on ordinal ranking of combinations of those variables which influence individual welfare.

(d) Interpersonal comparisons of utility involving value judgments are freely permissible.

Explanation:

Given these assumptions, it is possible to depict the social welfare function on a diagram by drawing a series of ‘well-behaved social indifference curves’ with commodities measured along the two axes. Each indifference curve shows various distributions of utility among individuals which have the same level of social welfare. Such curves help the policy maker to find out whether a particular policy brings an improvement or not. If a change moves individuals to a higher indifference curve, social welfare is said to have increased.

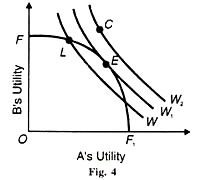
The social welfare function is explained diagrammatically in terms of Figure 4. FF₁ is the utility frontier which represents the boundary of all utility combinations possible with the given resources of the economy. It is an envelope of a number of overlapping utility possibility curves. W, W₁ and W₂ form the family of curves representing the social welfare function. Each welfare curve shows a locus of welfare combinations of the utilities of two individuals A and В who are indifferent.

Each welfare curve represents a level of social welfare. The welfare curve W₁ depicts a higher level of social welfare than curve W and W₂ higher level than W_1. The point of maximum social welfare or optimum position is one where the utility frontier curve FF, is tangent to the welfare curve. In the figure, point E clearly represents the situation of maximum social welfare or the bliss point.

Within the constraints of given technology and fixed quantities of inputs, of all the welfare combinations open to society, E has the highest social value. Point i is on a lower welfare curve W and represents a lower level of social welfare, whereas point С on the W₂ curve is beyond the utility frontier FF, of the society. Thus point E represents maximum social welfare.

It’s Criticism:

The assumptions of the Bergson criterion have tended to make the social welfare function ‘as broad and empty as language itself —and as necessary,’ according to Samuelson. Others have hailed it ‘as a major contribution to welfare economics’, while for Dr. Little, it completes the formal mathematical system of welfare economics. Scitovsky regards it as ‘completely general’.

Baumol judges it as ‘right, not very helpful,’ while to Robertson it is a ‘vast partly coloured mathematical balloon’. Paul Streeten praises it as ‘a device which is supposed to purify economic investigation of all vestiges of unscientific matter’. In the intermingling of these approvals and half- approvals are to be found the following limitations of these criterions.

(1) Not Applicable either to a Totalitarian State or a Democratic One:

The social welfare function is analogous to the individual consumer’s utility function which provides a ranking-‘from the point of view of a benevolent despot, or a complete egoist, or all men of goodwill’— of alternative utility levels enjoyed by different individuals. But Little regards it as inapplicable in a totalitarian state and more so in a democratic one “where there would be as many (vague) welfare functions as there are individuals. It can be regarded only as ‘a formal device necessary to a perfectly general abstract system of welfare,’ which bears no relation to practical policy.”

(2) Construction of Welfare Function Difficult:

Another difficulty arises with regard to the construction and shape of the welfare function. The social welfare function is constructed by aggregating each individual’s preferences. But the problem is whether individual preferences should be given equal or different weights. This makes the construction of the social welfare function a difficult task.

(3) Arbitrary and Imaginary:

The representation of the social welfare function in terms of either equations or social an indifference curve does not help solve the problem because individual welfare functions cannot be known. Therefore, all equations and curves representing the social welfare function are arbitrary and imaginary.

(4) The Concept of “Maximum ‘ without any Empirical Significance:

According to Little, “The maximum is a concept without any possible empirical significance, and therefore it seems preferable not to use it. It is more meaningful to derive the ‘optimum’ conditions as sufficient conditions for an improvement with- out attempting to define a maximum position.”

(5) Contradictory Results:

Prof. Arrow points out that the construction of a social welfare function on the basis of ordinal preferences leads to contradictory results if individuals are required to make choices from among more than two alternatives.

(6) Not Helpful in Solving Problems:

According to Prof. Baumol, “The social welfare function does not come equipped with a kit and a set of instructions for collecting the welfare judgments which it requires.” Thus it is not of much help in solving the main problems of welfare economics.

Criterion of Welfare # 4. Arrow’s Impossibility Theorem:

Bergson in his social welfare function showed that a social ranking of alternative economic situations can be made only by making interpersonal comparisons of utility that are incorporated in such a function. But the question arises whether it is an imposed function reflecting the tastes of a dictator or the preferences of the society in some democratic way. K.J. Arrow in his Social Choice and Individual Values has demonstrated the impossibility of obtaining the social welfare function even if individual preferences are consistent. He suggests five minimum conditions or criteria which social choices must satisfy in order to reflect preferences of individuals.

They are as follows:

1. Collective Rationality:

All possible alternatives must be derived from social choices which, in turn, must be based on rationality. The rule for making a social choice can be derived from an ordering of all possible alternatives open to society. This ordering must obey two conditions, of consistency and transitivity.

Consistency refers to the requirement that individuals have preferences that are fully defined, i.e. every alternative is ranked in relation to every other. Second, social choices must satisfy the condition of transitivity. If an individual prefers X to Y and Y to Z, then he must prefer J to Z. Thus social preferences, like individual preferences, must be completely ordered.

2. Responsiveness to Individual Preferences:

Social choices must be directly related to individual preferences. It implies that social choices must change in the same direction as individual choices. Individual choices must be derived within the society. But it is not possible to derive such alternatives which affect the socially desirable alternatives.

3. Non-imposition:

Social choices must not be imposed by customs or from outside the society. It must be derived from individual preferences. For instance, if the majority of individuals do not prefer to B, then the society should not follow it.

4. Non-dictatorship:

Social choices must not be dictatorial. They must not be imposed by one individual within the society. In other words, social choices must not be based on any single individual’s ordering.

5. Independence of Irrelevant Alternatives:

Social choices must be independent of irrelevant alterna­tives. In other words, if any one alternative is excluded, it will not affect the ranking of other alternatives. Arrow demonstrates that it is not possible to satisfy all these five conditions and obtain a transitive social choice for each set of individual preferences without violating at least one condition.

In other words, social choice is inconsistent or undemocratic because no voting system allows these five conditions to be satisfied. This has come to be known as the Arrow Impossibility Theorem. To illustrate Arrow’s general impossibility theorem, suppose there are three individuals A, B and C in a society. They are asked to rank three alternative situations X, Y and Z They vote by writing number 3 for their first choice, 2 for their second choice and 1 for their third choice. Suppose the voting pattern is as shown in Table 1.

The table shows that each individual has consistent preferences. A prefers X to Y, and Y to Z and hence X to Z. B prefers Y to Z, and Z to X, and hence Y to X. C prefers Z to X, and X to Y and hence Z to X But the majority voting leads to intransitive social patterns.

Two individuals A and C prefer X to Y. Two individuals A and B prefer Y to Z. Nonetheless, B and C prefer Z to X. Hence the majority prefers X to Y and Y to Z, but it also prefers Z to X. This is illustrated in Figure 5 which shows a multiple-peaked pattern. This explains the paradox of the majority rule which is inconsistent with those of individuals composing the majority.

Thus Arrow shows that the use of the democratic process of voting leads to a contradictory welfare criterion. “This ‘voting paradox’ explored by Prof. Arrow, comes as a shock to one’s faith in electoral democracy,” according to Prof. Musgrave. “Fortunately the paradox does not imply that majority rule cannot work. Rather, the conclusion is that for majority rule to give non-arbitrary results, the preference structure of individuals must be typically single-peaked.” By single-peaked preference patterns, Musgrave means, where there is an absence of voters with “extremist” preference patterns.

It’s Criticism:

Arrow’s general impossibility theorem has been criticised by Samuelson, Little and other welfare econo­mists on the following grounds:

1. Not related to Social Welfare Function:

According to Little, Arrow’s negative conclusions have no relevance in welfare economics. His impossibility theorem relates to a decision making process and not to a social welfare function.

2. No Solution of Interpersonal Comparisons:

Mishan opines that Arrow fails to solve the problem of interpersonal comparison of utility in his search for a satisfactory social welfare function. Rather, his method of majority rule involves interpersonal comparisons. If a majority prefers X to Y, then the majority decision in favour of X means that X is preferred to Y only if the aim is to maximise utility and the choice of one individual has equal utility with that of another.

3. Mathematical Politics:

Samuelson is of the view that Arrow has proved the impossibility of “a political constitution function” which would be able to resolve any interpersonal differences brought to it while at the same time satisfying certain reasonable and desirable axioms. Thus the Arrow result is a basic theorem of what Samuelson calls “mathematical politics”.

4. Social Choice not the only Alternative.

Baumol shows that “Arrow’s requirements are more strict than they seem at first view and that inconsistent or “undemocratic” social choice-making is not really the only alternative.

5. Majority Voting Pattern Unrealistic:

Moreover, the Arrow theorem is based on the assumption of a majority voting pattern which does not take into consideration the possibility of a voting system that requires unanimity and permits buying and selling of votes.

Conclusion:

Despite these criticisms, J.M. Buchanan holds that “the alternative to majority voting may be failure to make collective choices and there is no reason to believe that this is a preferred course of action. Voting is a means by which collective choices are made, and imperfections in the voting mechanism hardly seem an adequate reason to discard the system.”