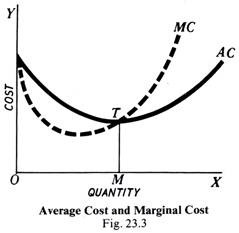
Curves can be drawn to represent costs. The marginal cost (MC) and the average cost (AC) are shown in the following diagram (23.3).
OX and OY are two axes, along OX is shown the quantity produced and along OY the cost.
It will be seen that as output is increased, both average cost (AC) and marginal cost (MC) fall, but MC is below AC, i.e., marginal cost is less than the average cost.
The fall is due to the economies of scale. But beyond a point (M), i.e., when output is expanded too much, both AC and MC start rising and now MC is above AC, i.e., the marginal cost is greater than the average cost. That is why MC cuts AC from below at its lowest point.
ADVERTISEMENTS:
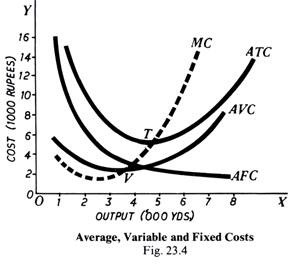
There are two types of other cost curves. They are the Average Variable Cost Curve and the Average Fixed Cost Curve. The average variable cost is obtained by dividing the total variable cost by the number of units produced. Average fixed cost is obtained by dividing the total fixed cost by the total units of output. The total fixed cost being fixed for all units of output, AFC is a falling curve in the shape of a rectangular hyperbola.
The average variable and average fixed cost curves can be represented as below (Fig. 23.4). OX and OY are the two axes. The output is represented along OX and cost along OY; AFC curve represents average fixed cost. AVC curve represents average variable cost, ATC curve represents average total cost (i.e., total of AFC and AVC and is called AC, i.e., average cost). MC curve represents marginal cost.
It is clear that as output is increased, the cost per unit decreases due to various internal economies. That is why AFC, AVC and hence ATC all start falling. But if the output is expanded beyond proper limit, diseconomies will result, and all these costs will go up, which is shown by the rising portions of these curves. This is the third stage of the Law of Variable Proportions.
It will be seen that AFC continues to fall, it never rises, and rather it helps AVC to fall too for some time, but after some time AVC starts rising quickly and raises ATC too. MC and AVC fall and rise at the same time. But it is to be carefully noticed that when MC and AVC are falling, MC is below AVC, but when they rise, MC is above AVC. When AVC is constant, MC is equal to it. In other words, MC cuts AVC and ATC at their lowest points.