4 Strategies of the Game Theory – Explained!

In the game theory, different players adopt different types of strategies on the basis of the outcome, which is obtained by adopting the strategy.

For instance, the player may adopt a single strategy every time as it provides him/her maximum outcome or he/she can adopt multiple strategies.

Apart from this, a player may also adopt a strategy that provides him/her minimum loss. Therefore on the basis of outcome, the strategies of the game theory are classified as pure and mixed strategies, dominant and dominated strategies, minimax strategy, and maximin strategy. Let us discuss these strategies in detail.

1. Pure and Mixed Strategies:

In a pure strategy, players adopt a strategy that provides the best payoffs. In other words, a pure strategy is the one that provides maximum profit or the best outcome to players. Therefore, it is regarded as the best strategy for every player of the game. In the previously cited example (Table-1), the increase in the prices of organizations’ products is the best strategy for both of them.

This is because if both of them increase the prices of their products, they would earn maximum profits. However, if only one of the organization increases the prices of its products, then it would incur losses. In such a case, an increase in prices is regarded as a pure strategy for organizations ABC and XYZ.

On the other hand, in a mixed strategy, players adopt different strategies to get the possible outcome. For example, in cricket a bowler cannot throw the same type of ball every time because it makes the batsman aware about the type of ball. In such a case, the batsman may make more runs.

However, if the bowler throws the ball differently every time, then it may make the batsman puzzled about the type of ball, he would be getting the next time.

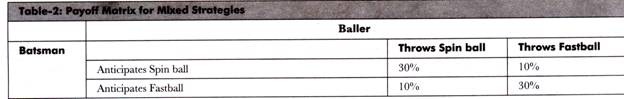
Therefore, strategies adopted by the bowler and the batsman would be mixed strategies, which are shown ion Table-2:

In Table-2, when the batsman’s expectation and the bowler’s ball type are same, then the percentage of making runs by batsman would be 30%. However, when the expectation of the batsman is different from the type of ball he gets, the percentage of making runs would reduce to 10%. In case, the bowler or the batsman uses a pure strategy, then any one of them may suffer a loss.

Therefore, it is preferred that bowler or batsman should adopt a mixed strategy in this case. For example, the bowler throws a spin ball and fastball with a 50-50 combination and the batsman predicts the 50-50 combination of the spin and fast ball. In such a case, the average hit of runs by batsman would be equal to 20%.

This is because all the four payoffs become 25% and the average of four combinations can be derived as follows:

0.25(30%) + 0.25(10%) + 0.25(30%) + 0.25(10%) = 20%

However, it may be possible that when the bowler is throwing a 50-50 combination of spin ball and fastball, the batsman may not be able to predict the right type of ball every time. This would decrease his average run rate below 20%. Similarly, if the bowler throws the ball with a 60-40 combination of fast and spin ball respectively, and the batsman would expect either a fastball or a spin ball randomly. In such a case, the average of the batsman hits remains 20%.

The probabilities of four outcomes now become:

Anticipated fastball and fastball thrown: 0.50*0.60 = 0.30

Anticipated fastball and spin ball thrown: 0.50*0.40 = 0.20

Anticipated spin ball and spin ball thrown: 0.50*0.60 = 0.30

Anticipated spin ball and fastball thrown: 0.50*0.40 = 0.20

When we multiply the probabilities with the payoffs given in Table-2, we get

0.30(30%) + 0.20(10%) + 0.20(30%) + 0.30(10%) = 20%

This shows that the outcome does not depends on the combination of fastball and spin ball, but it depends on the prediction of the batsman that he can get any type of ball from the bowler.

2. Dominant and Dominated Strategies:

A dominant strategy is the one that is best for an organization (player) and is not influenced by the strategies of other organizations (players). Let us understand the dominant strategy with the help of the example given in Table-1. Suppose organizations ABC or XYZ adopt a dominant strategy.

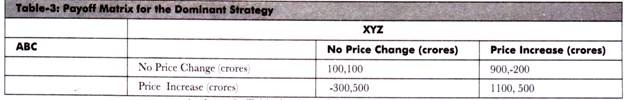
In such a case, their payoff matrix is shown in Table-3:

As shown in Table-3, when ABC is not making any change in prices, then XYZ has also not changed its prices. This would results as the best strategy of XYZ. However, when ABC has increased its prices, then XYZ would earn profit of Rs. 300 crores by keeping its prices constant. When XYZ increases its prices, it would earn Rs. 500 crores.

Therefore, it is better for XYZ to make its price constant so that it can earn more. The dominant strategy- for XYZ is to keep the prices of its products constant. On the other hand, the dominant strategy- of ABC would also be to keep the price constant. This is because ABC would incur losses if it increases the prices of its products.

While analyzing games, the player who has adopted the dominant strategy is identified and then the strategies of other players in the game are judged on the basis of the dominant strategy. However, the existence of the dominant strategy in every game is not possible.

On the other hand, a dominated strategy is the one that provides players the least payoff as compared to other strategies in a game. In the analysis of the game theory, dominated strategies are identified so that they can be eliminated from the game. Let us understand the dominated strategy with the help of an example.

Suppose in a football match, the aim of offense team is to maximize its goals, while that of defense team is to minimize the offense’s goal. Now, assume that there are only two plays left and the ball is with the offense team.

In this case, the offense team would adopt two strategies; one is to run and another is to pass. On the other hand, the defense team would have three strategies; one is to defend against running, defend against pass through line-backers and defend against pass through quarterback blitz.

Table-4 shows the outcomes of the strategies adopted by offense and defense team:

In Table-4, the numerical value represents the goals made by the offense team. In this case, neither offense nor defense team have a dominant strategy. However, the defense team does have one dominated strategy that is quarterback blitz.

Either in case of defending run or pass, quarterback blitz strategy would yield more goals to the offense team. Therefore, the defense team should avoid quarterback blitz strategy. Dominated strategy helps in making the analysis of game easier by reducing the number of options.

3. Maximin Strategy:

As we know, the main aim of every organization is to earn maximum profit. However, in the highly competitive market, such as oligopoly, organizations strive to reduce the risk factor. This is done by adopting the strategy that increases the probability of minimum outcome. Such a strategy is termed as maximin strategy.

In other words, maximin strategy is the one in which a player or organization maximizes the probability of minimum profit so that the degree of risk can be reduced. Let us understand the maximin strategy with the help of an example. Suppose two organizations, A and B, want to launch a new product in a duopoly market.

The outcomes for these two organizations are shown in Table-5:

In Table-5, it is assumed that the main motive of both the organizations is to maximize their profits. Let us first analyze the outcome of organization B. Organization B would earn profit of Rs. 4 crores when both the organizations, A and B, launch a new product However, if only organization A launches a new product, then the profit of organization B would be Rs. 6 crores.

However, if organization B launches a new product, then it would earn profit of Rs. 4 crores. Therefore, the minimum gain of organization B is Rs. 4 crores after launching a new product. Similarly, the minimum gain of A is Rs. 4 crores by launching a new product. Maximin strategy is not used only for profit maximization problems, but it is also used for restricting the unrealistic and highly unfavorable outcomes.

For applying the maximin strategy, firstly, an organization needs to identify the minimum output or profit that it would get from a particular strategy. Table-5 shows that the minimum output for organization A is Rs. 6 crores when it does not launch a new product. However, if it launches a new product, the minimum output would be Rs. 4 crores.

On the other hand, organization B also has the same amount of profit in both the cases. Now, both the organizations, A and B, would find out the strategy that would yield them maximum of the minimum output. In the present case, for both the organizations, A and B, it would be better if they do not launch any new product to yield maximum profit.

4. Minimax Strategy:

Minimax strategy is the one in which the main objective of a player is to minimize the loss and maximize the profit. It is a type of mixed strategy. Therefore, a player can adopt multiple strategies. It can be applied to complex as well as simple decision-making process. Let us understand the minimax strategy with the help of an example.

Suppose Mr. Ram wants to manufacture cream biscuits. For this, he selected three flavors, namely strawberry, chocolate, and pineapple, which he denoted with A, B, and C respectively He wants to select one of the flavors to produce cream biscuits and introduce them in the market on the basis of their demand.

He needs to predict the future events that can occur from the options he has selected. These future events are termed as the states of nature in decision analysis. The states of nature selected by Ram with respect to demand are high demand, medium demand, and low demand.

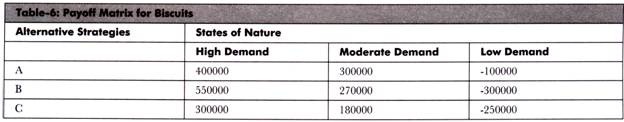
The payoff matrix for biscuits is shown in Table-6:

Here, we are assuming that Mr. Ram adopts minimax strategy. Now, if he selects strategy A in a high demand market, then he would incur a loss of Rs. 150000. This is because he has not selected the strategy B that would yield maximum payoff of Rs. 550000.

In such a case, he would determine the maximum loss for each alternative and then select the alternative that would give minimum loss. Among each state of nature, the highest payoff is selected and subtracted from all other values in the state of nature.

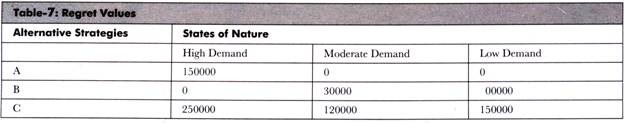
Table-7 shows the loss or regret values of A, B, and C strategies:

In Table-7, the maximum regret in each state of nature is highlighted with blue color. Among the highlighted regret values, strategy C has the least regret value of Rs. 120000. Therefore, Ram would select the strategy- C or pineapple flavor to produce biscuits.